Brain Teaser: Do You Know the Rule of the Square?

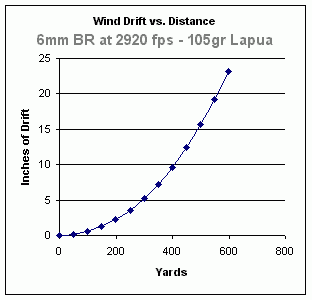Horizontal Wind-Drift vs. Distance
OK, here’s a challenge question for you.
Let’s see if you get it right.

Q: If the wind is blowing 10 mph from 9 o’clock and if my horizontal wind deflection is 0.7 inches at 100 yards, what is the horizontal drift at 1000 yards?

You may be thinking, “Well, since the target is ten times more distant, the wind-drift should be around 7 inches, maybe a little more since the bullet will be slowing down.” That sounds reasonable, right?

WRONG.

As you move from near to far, the increase in lateral deflection (from a 90° crosswind) is (roughly speaking) a function of the square of the multiple of distance. If your target is two times farther away, you use the square of two, namely four. If your target is five times farther away, you use the square of five, or twenty-five. In this example, the increased wind drift (from 100 to 1000 yards) is at least 0.7″ times (10 X 10) — over 70 inches (give or take a few inches depending on bullet type). We call that the Rule of the Square. This Rule lets you make a quick approximation of the windage correction needed at any yardage.

Precision Shooting and the Rule of the Square
I was going through some back issues of Precision Shooting Magazine and found many references to the Rule of the Square. This made me curious — I wondered how well the Rule really stacked up against modern ballistics programs. Accordingly, I ran some examples through the JBM Ballistics Trajectory Calculator, one of the best web-based ballistics programs. To my surprise, the Rule of the Square does a pretty good job of describing things.

EXAMPLE ONE — .308 Win (100 to 400 Yards)
For a 168gr Sierra MK (.308), leaving the muzzle at 2700 fps, the JBM-predicted values* are as follows, with a 10 mph, 9 o’clock crosswind (at sea level, 65° F, Litz G7 BC):

Drift at 100: 0.8 MOA (0.8″)
Drift at 200: 1.6 MOA (3.3″)
Drift at 400: 3.4 MOA (14.4″)

Here you can see how the Rule of the Square works. The rule says our drift at 200 yards should be about FOUR times the drift at 100. It the example above, 0.8″ times 4 is 3.2″, pretty darn close to the JBM prediction of 3.3″. Quoting Precision Shooting: “Note that the deflections at 100 yards are typically a quarter of those at 200; lateral deflections increase as the square of the range”. Precision Shooting, June 2000, p. 16.

EXAMPLE TWO — .284 Win (100 to 1000 Yards)
For a .284 Win load, with the slippery Berger 180gr Target Hybrids, the Rule of the Square still works. Here we’ll input a 2750 fps velocity, Litz G7 BC, 10 mph, 9 o’clock crosswind, (same 65° temp at sea level). With these variables, JBM predicts:

Drift at 100: 0.5 MOA (0.5″)
Drift at 500: 2.5 MOA (13.3″)
Drift at 1000: 5.9 MOA (61.3″)

Again, even with a higher BC bullet, at 1000 yards we end up with something reasonably close to the 100-yard deflection (i.e. 0.5″) multiplied by (10×10), i.e. 50 inches. The Rule of the Square alerts you to the fact that the effects of crosswinds are MUCH greater at very long range. In this example, our JBM-calculated drift at 1000 is 61.3″ — that’s over 100 times the 100-yard lateral drift, even though the distance has only increased 10 times.

Note that, even with a 5 mph 90° sidewind, the “Rule of the Square” still applies. The 1000-yard lateral deflection in inches is still over 100 times the lateral deflection at 100 yards.

Why This All Matters (Even in the Age of Smartphones)
Now, some would say, “Why Should I Care About the Rule of the Square? My iPhone has a Ballistics App that does all my thinking for me”. Fair enough, but knowledge of this basic Rule of the Square enables a shooter to make an informed guess about necessary windage even without a come-up sheet, as long as he knows the distance AND can fire a sighter at 100 or 200 yards as a baseline.

For example, if I see empirically that I need 1″ windage correction at 100 yards, then I know that at 600 yards I need at least roughly (6 x 6 x 1″) or 36 total inches of drift correction, or 6 MOA. (To be precise, 1 MOA = 1.047″ at 100 yards). I can figure that out instantly, even without a ballistics chart, and even if my Smartphone’s battery is dead.

*Values shown are as displayed on the JBM-figured trajectory tables. The numbers can be slightly imprecise because JBM rounds off to one decimal place for both inches and MOA.