Ballistics Linguistics: Bullet “Caliber of Ogive” Defined

This article, which originally appeared in the Sierra Bullets Blog, provides a new terminology that helps describe the geometry of bullets. Once you understand the meaning of “Caliber(s) of Ogive”, you can quickly evaluate potential bullet performance by comparing listed Caliber of Ogive numeric values.

by Mark Walker, Sierra Bullets New Product Development Manager
During one of our recent product releases, we listed the “caliber of ogive” of the bullet in the product description. While some understood what that number meant, it appears that some are not aware of what the number is and why it is important. In a nutshell, the “caliber of ogive” number will tell you how sleek the front end of the bullet is. The higher the number is, the sleeker the bullet. It also makes it easy to compare the ogives of different caliber bullets. If you want to know if a certain .308 caliber bullet is sleeker than a 7mm bullet, simply compare their “caliber of ogive” numbers.

So exactly how do you figure “caliber of ogive”? If you look at the drawing of the .30 caliber 175 gr HPBT bullet #2275 (at top), you will see that the actual radius of the ogive is 2.240. If you take that 2.240 ogive radius and divide by the diameter (or caliber) of the bullet you would get 7.27 “calibers of ogive” (2.240 ÷ .308 = 7.27). (See top photo).

In a nutshell, the “caliber of ogive” number will tell you how sleek the front end of the bullet is. The higher the number is, the sleeker the bullet.

Next let’s look at the print (below) of our 6.5mm 142gr HPBT #1742 bullet for comparison. The actual radius of the ogive is 2.756. Like with the .30 caliber 175 gr HPBT bullet #2275, if you divide 2.756 by the diameter (or caliber) of the bullet you get 10.44 “calibers of ogive”.

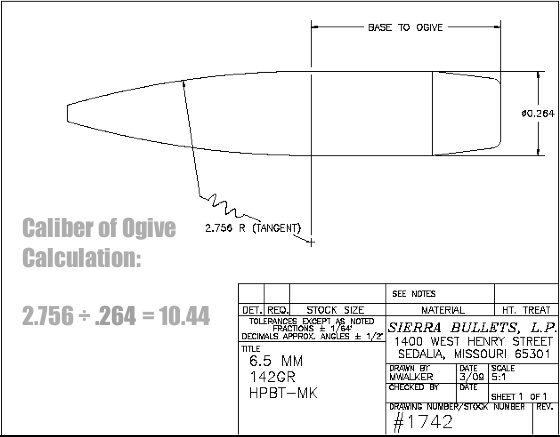
As most people know, it has been determined through testing that the 6.5mm 142gr HPBT #1742 has a significantly higher ballistic coefficient than the .30 caliber 175 gr HPBT bullet #2275. However by simply comparing the “caliber of ogive” number of each bullet you can easily see that the 6.5mm 142 gr HPBT #1742 is significantly sleeker than the .30 caliber 175 gr HPBT bullet #2275 even without firing a shot.

Some people would say why not just compare the actual ogive radius dimensions instead of using the “caliber of ogive” figure. If we were comparing only bullets of the exact same diameter, then that would be a reasonable thought process. However, that idea falls apart when you start trying to compare the ogives of bullets of different diameters. As you can see with the two bullets presented above, if we compare the actual ogive radius dimensions of both bullets the difference is not much at all.

However, once again, testing has shown that the 6.5mm 142 gr HPBT #1742 has a significantly higher BC. The only way that this significant increase shows up, other than when we fire the bullets in testing, is by comparing the “caliber of ogive” measurement from both bullets.

Hopefully this will help explain what we mean when we talk about “caliber of ogive” and why it’s a handy number to use when comparing bullets. This information will help you to make an informed decision the next time you are in the market to buy bullets.

Similar Posts:

• Tangent vs. Secant vs. Hybrid Ogive — Bryan Litz Explains
• Ogive No Jive — Litz Explains Tangent, Secant, and Hybrid Ogives
• Bullet Geometry Basics: Tangent, Secant, and Hybrid Ogives
• Tangent, Secant, Hybrid — Bullet Geometry Explained by Litz
• Bullet Geometry: Tangent, Secant, and Hybrid Ogives Explained

Tags: bullet, Hybrid, Ogive, Secant, Sierra Bullets, Tangent