Bullet RPM and Drag — How BC Changes with Bullet Spin Rates
You may not realize it… but to get the optimum BC from your bullets (i.e. the lowest aerodynamic drag), you must spin the bullets fast enough. Bullet drag increases (as expressed by lower BC) if the bullet spins too slowly. Bryan Litz of Applied Ballistics explains how BC changes with twist rates…
More Spin, Less Drag
In this article, we look at how twist rate and stability affect the Ballistic Coefficient (BC) of a bullet. Again, this topic is covered in detail in the Modern Advancements book. Through our testing, we’ve learned that adequate spin-stabilization is important to achieving the best BC (and lowest drag). In other words, if you don’t spin your bullets fast enough (with sufficient twist rate), the BC of your bullets may be less than optimal. That means, in practical terms, that your bullets drop more quickly and deflect more in the wind (other factors being equal). Spin your bullets faster, and you can optimize your BC for best performance.
Any test that’s designed to study BC effects has to be carefully controlled in the sense that the variables are isolated. To this end, barrels were ordered from a single barrel smith, chambered and headspaced to the same rifle, with the only difference being the twist rate of the barrels. In this test, 3 pairs of barrels were used. In .224 caliber, 1:9” and 1:7” twist. In .243 caliber it was 1:10” and 1:8”, and in .30 caliber it was 1:12” and 1:10”. Other than the twist rates, each pair of barrels was identical in length, contour, and had similar round counts. Here is a barrel rack at the Applied Ballistics Lab:
Applied Ballistics used multiple barrels to study how twist rate affects BC.
“The Modern Advancements series is basically a journal of the ongoing R&D efforts of the Applied Ballistics Laboratory. The goal of the series is to share what we’re learning about ballistics so others can benefit.” –Bryan Litz
Barrel twist rate along with velocity, atmospherics, and bullet design all combine to result in a Gyroscopic Stability Factor (SG). It’s the SG that actually correlates to BC. The testing revealed that if you get SG above 1.5, the BC may improve slightly with faster twist (higher SG), but it’s very difficult to see. However, BC drops off very quickly for SGs below 1.5. This can be seen in the figure below from Modern Advancements in Long Range Shooting.
The chart shows that when the Gyroscopic Stability Factor (SG) is above 1.5, BC is mostly constant. But if SG falls below 1.5, BC drops off dramatically.
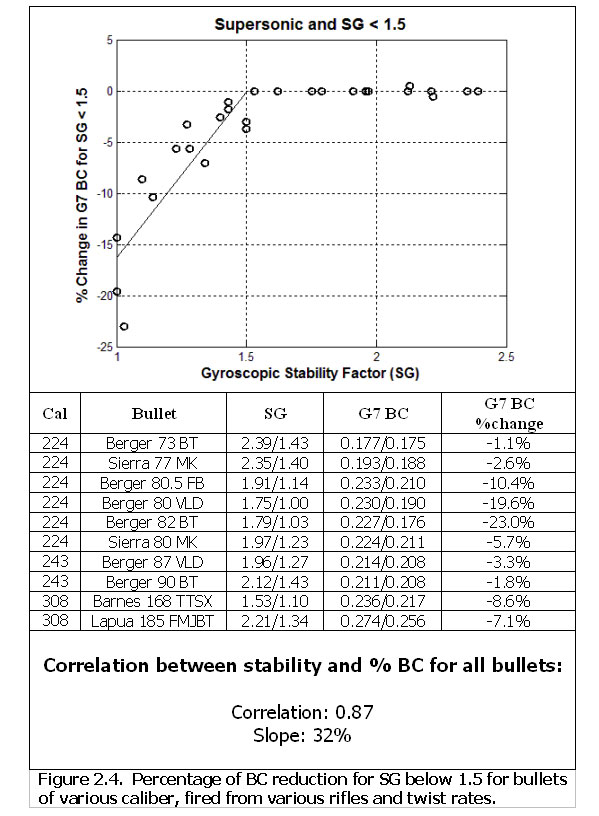
Note that the BC drops by about 3% for every 0.1 that SG falls below 1.5. The data supports a correlation coefficient of 0.87 for this relationship. That means the 3% per 0.1 unit of SG is an accurate trend, but isn’t necessarily exact for every scenario.
It’s a common assumption that if a shooter is seeing great groups and round holes, that he’s seeing the full potential BC of the bullets. These tests did not support that assumption. It’s quite common to shoot very tight groups and have round bullet holes while your BC is compromised by as much as 10% or more. This is probably the most practical and important take-away from this test.
To calculate the SG of your bullets in your rifle, visit the Berger Bullets online stability calculator. This FREE calculator will show you the SG of your bullets, as well as indicate if your BC will be compromised (and by how much) if the SG is below 1.5. With the stated twist rate of your barrel, if your selected bullet shows an SG of 1.5 (or less), the calculator will suggest alternate bullets that will fully stabilize in your rifle. This valuable online resource is based directly on live fire testing. You can use the SG Calculator for free on the web — you don’t need to download software.
Learn More About SG and BC
This article is just a brief overview of the interrelated subjects of twist rate, Gyroscopic Stability, and BC. The coverage of twist rates in Modern Advancements in Long-Range Shooting is more detailed, with multiple live fire tests.
Other chapters in the book’s twist rate section include:
· Stability and Drag – Supersonic
· Stability and Drag – Transonic
· Spin Rate Decay
· Effect of Twist rate on Precision
Other sections of the book include: Modern Rifles, Scopes, and Bullets as well as Advancements in Predictive Modeling. This book is sold through the Applied Ballistics online store. Modern Advancements in Long Range Shooting is also available in eBook format in the Amazon Kindle store.
Similar Posts:
- New Spin on BC — How Barrel Twist Rates Affect Bullet Drag
- How Ballistic Coefficent Varies with Twist Rate (Stabilization)
- Berger Updates Free Online Bullet Stability Calculator
- Optimize Bullet RPM with Berger Twist Rate Stability Calculator
- Berger Twist Rate Stability Calculator — Optimize Bullet RPM
Share the post "Bullet RPM and Drag — How BC Changes with Bullet Spin Rates"
Tags: ballistics, Barrel Twist, BC, Drag Factor, RPM, Spin Rate
This was one of the best article i have read and learn from. Thanks.
If I understand correctly, the issue is that a bullet not spinning rapidly enough does not have enough gyroscopic force to keep the longitudinal axis of the bullet aligned with the axis of flight. The bullet will thus end up with a higher drag coefficient because the nose is precessing about the axis of flight, resulting in a different coefficient of drag than would be the case if it were precisely aligned aerodynamically with the axis of flight. In addition, it most likely would also present a greater frontal area as the yaw-induced cross section increased (i.e. the bullet flew more and more misaligned, or “sideways”), which would increase drag further. Finally, if a bullet is not aligned with the axis of flight, the Magnus Effect would impose a lift vector, from the instantaneous “virtual crosswind” created by the lateral component of wind speed introduced by the forward “slew” of the bullet created by its yaw, perpendicular to the instantaneous angle of slew, which would create a corkscrew flight pattern.
One would think that the higher spin rate would introduce more aerodynamic turbulence and rotational drag, but if so, it seems it must be more than overcome by the alignment effects. I wonder if this phenomenon is measured directly – for example, by doppler radar – or inferentially – for example by drift, or group size, both of which could be compromised if the bullet path were not linear, but rather “corkscrew” in trajectory. At what point, I wonder, would increasing spin introduce secondary destabilizing effects from bullet imperfections, like voids/density variation that would introduce eccentricity in flight (spinning about the mass centerline/”center of gravity” instead of the geometric/bore axis centerline)?